ACTIVE SOUND CONTROL

This research was supported by EPSRC (project GR/T26832/01, 01/04/2005-31/07/2008) and performed in co-operation with the Acoustics Research Centre at Salford University. The main project task consists of theoretical development and experimental implementation of a new technique for active shielding of noise. The approach provides a new alternative to current active noise control techniques that require sampling of the domain of the acoustic field. The principal innovation of the approach includes the capabilities of volume noise cancellation and automatic separation of incoming (“friendly”) and outgoing (“adverse”) components.

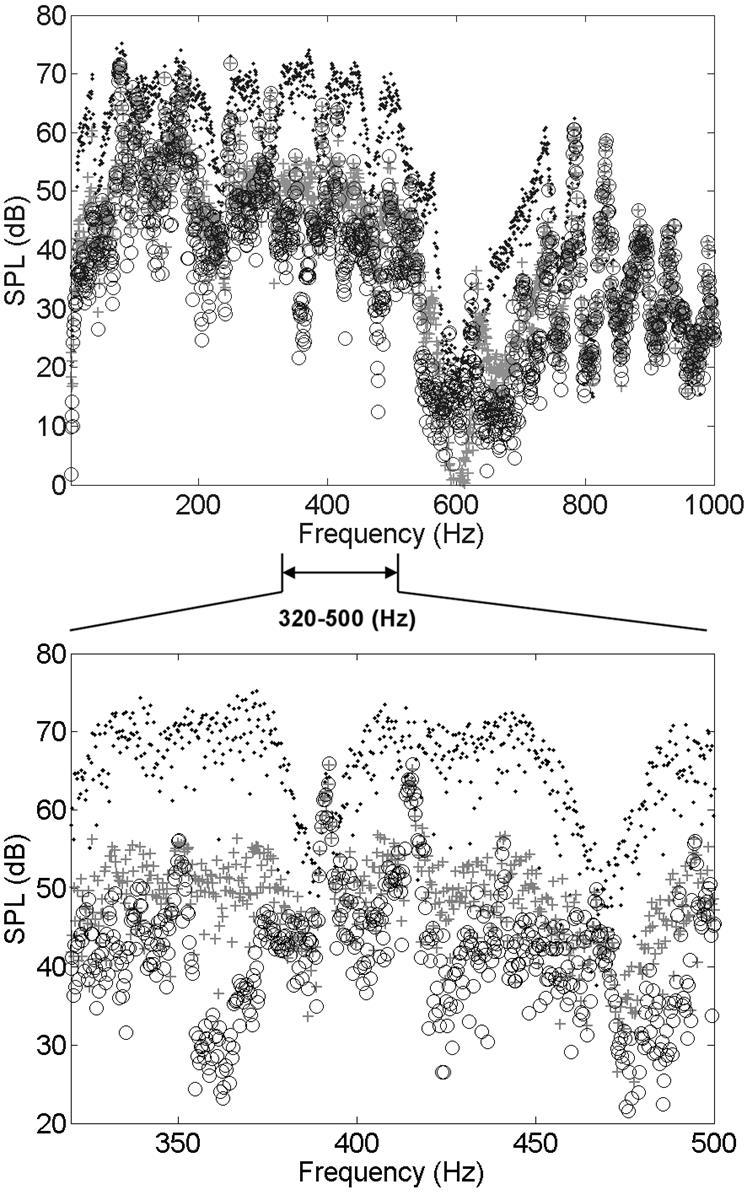
Experimental implementation of the theoretical solution. ○: wanted sound pressure, ♦: the sound pressure of noise and wanted sound without control, and +: total sound pressure of noise and wanted sound, and controls. Bottom graph is a zoom-in of the result in the 320-500Hz frequency range.

1. Ryaben'kii, V.S., and Utyuzhnikov, S.V., “Active shielding model for hyperbolic equations”, IMA Journal of Applied Mathematics, 2006, 71 (6): 924-939.

2. Ryaben'kii, V.S., and Utyuzhnikov, S.V., “Differential and finite-difference problems of active shielding”, J. Applied Numerical Mathematics, 2007, 57 (4): 374-382.

3. Ryaben'kii, V.S., Tsynkov, S.V., and Utyuzhnikov, S.V., “Inverse source problem and active shielding for composite domains”, J. Applied Mathematics Letters, 2007, 20 (5): 511-515.

4. Ryaben’kii, V.S., Utyuzhnikov, S.V., and Turan, A.A., “On the application of difference potential theory to active noise control”, J. Advances in Applied Mathematics, 2008, 40 (2): 194-211.

5. Ryaben’kii, V.S., Tsynkov, S.V., and Utyuzhnikov, S.V., “Active control of sound with variable degree of cancellation”, J. Applied Mathematics Letters, 2009, 22 (12): 1846-1851.

6. Utyuzhnikov, S.V., “Generalized Calderon-Ryaben’kii’s potentials”, IMA J. of Applied Mathematics, 2009, 74 (1): 128-148.

7. Utyuzhnikov, S.V., “Active wave control and generalized surface potentials”, J. Advances in Applied Mathematics, 2009, 43 (2): 101-112.

8. Lim, H., Utyuzhnikov, S. V., Lam, Y. W., Turan, A., Avis, M. R., Ryaben'kii, V. S., Tsynkov, S. V., “An experimental validation of the active noise control methodology based on difference potentials”, AIAA J., 2009, 47 (4): 874-884.

9. Utyuzhnikov, S.V., “Nonlinear Problem of Active Sound Control”, J. of Computational and Applied Mathematics, 2010, 234 (1): 215-223.

10. Utyuzhnikov, S V., "Non-stationary problem of active sound control in bounded domains". J. of Computational and Applied Mathematics, 2010, 234 (6): 1725-1731.

11. Lim, H., Utyuzhnikov, S. V., Lam, Y. W., Turan, A., "Multi-domain active sound control and noise shielding", J. of Acoustical Society of America, 2011, 129 (2): 717-725.

12. Lim H., Utyuzhnikov S V, Lam Y.W., Kelly L.. "Potential-based methodology for active sound control in three dimensional settings", J. of the Acoustical Society of America, 2014, 136 (3): 1101-1111.

13. Ntumy, E., and Utyuzhnikov, S.V., “Active Sound Control in 3D Bounded Regions”, J. Wave Motion, 2014, 51 (2): 284-295.

14. Utyuzhnikov, S.V., “Real-time Active Wave Control with Preservation of Wanted Field”, IMA J. Applied Mathematics, 2014, 79: 1126-1138.

15. Комментарии к препринту B.С. Рябенького от 2016 г.N 27,” Активная защита акустического поля желательных источников от внешнего шума в реальном времени”.

16. Utyuzhnikov, S.V., “A Practical Algorithm for Real-time Active Sound Control with Preservation of Interior Sound”, Computers & Fluids, 2017, 157: 175-181.