Research Interests
Giant Amazonian waterlilies
The giant Amazonian waterlily (genus Victoria) produces the largest floating leaves of all plants.
We studied how the structural form of the vasculature system underpins gigantism in these extraordinary leaves.
and infer how this unique form of leaf gigantism evolved.
Specifically, by means of mechanical testing and geometrical modelling, we found that the bending resistance of the Amazonian waterlily is considerably higher than of an elastic floating sheet of the same amount of material.
Our analysis suggests that the unique pattern of branching veins on the underside of the Victoria leaf provides structural support at an economical material cost and, as such, enables gigantism.
Considering the evolutionary history of the waterlily family, and the life history of Victoria in particular, we inferred that this multi-purpose system may have evolved to maximise photosynthesis and enable rapid growth in fast-drying pools, thereby conferring a selective advantage in an unstable environment.
Gigantic floating leaves occupy a large surface area at an economical material cost
F. Box, A. Erlich, J. H. Guan, and C. Thorogood Science Advances, 8(6), eabg3790 (2022), pdf
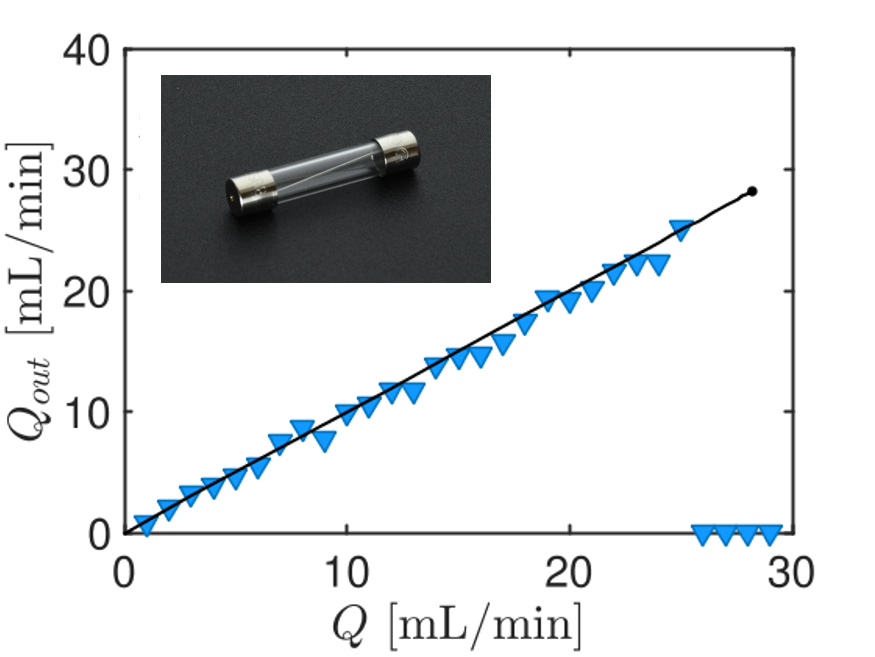
A fluidic fuse
In microfluidic devices, fluidic analogues of electrical circuit components provide opportunties for on-board actuation and can be rendered passive by using soft elastomeric features that respond to the flow.
The electronic circuit component so far overlooked happens to be one of the most ubiquitous – the humble fuse.
We demonstrate that fuse-like behaviour can be achieved in a fluidic circuit by integrating a soft slab of elastic into a channel wall.
Providing the soft slab is laterally confined, flow-induced deformation of the elastomer results in the growth of a bulge that can block the channel and interrupt the flow.
We study the bulging process using scaling analyses, numerical computations and experiments.
Crucially, we show that the bulge interrupts the flow through the channel when the flux exceeds a critical value that depends entirely on the material properties, and geometry, of the system.
Flow-induced choking of a compliant Hele-Shaw cell
F. Box, G. G. Peng, D. Pihler-Puzović, and A. Juel Proceedings of the National Academy of Sciences 117(48), 202008273 (2020), pdf
Mechanical metamaterials
The Poisson’s ratio is a measure of how much a material expands laterally when compressed axially.
Common experience tells us that most materials have a positive Poisson’s ratio, although some materials, like foams, contract laterally under compression and have a negative Poisson's ratio.
A negative Poisson's ratio behaviour can engineered into elastic, metal and plastic sheets through the introduction of a regular array of circular holes.
This unnatural macro-scale property is a consequence of the symmetry-breaking elastic buckling of the elements that constitute the micro-architecture (i.e. the struts that surround the holes).
which means that holey sheets made from hard materials with vastly different microscopic structures and macroscopic properties exhibit strikingly similar mechanical behavior when compressed.
Curiously, however, plastic deformation of hard, holey materials leads to a negative post-buckling stiffness not seen in their elastic counterparts.
Hard Auxetic Metamaterials
F. Box, C. Johnson and D. Pihler-Puzović, Extreme Mechanics Letters 100980 (2020) pdf
Dynamic compression of elastic and plastic cellular solids
F. Box, R. Bowman and T. Mullin, Applied Physics Letters 103, 151909 (2013)
Pattern switching in soft cellular solids under compression
T. Mullin, S. Wilshaw and F. Box, Soft Matter 8, 1747-1750 (2013)
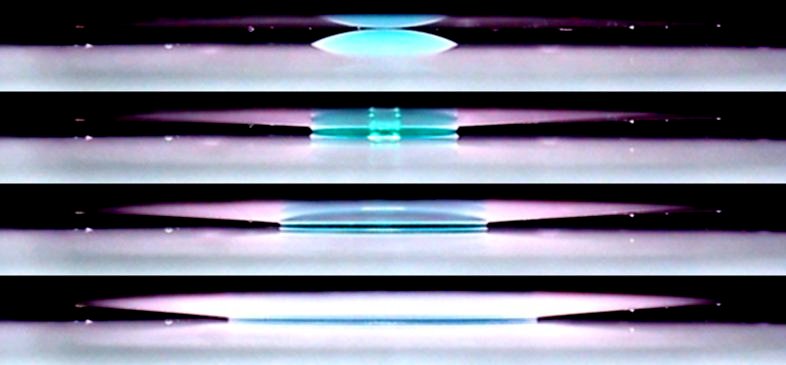
The fast and the flexible
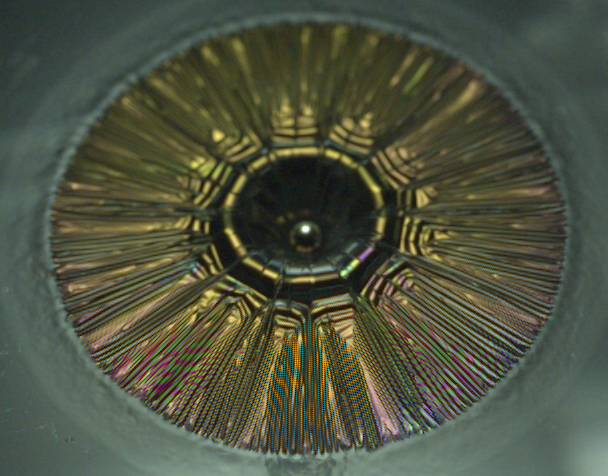
The figure-of-eight shape adopted by a loose rubber band is familiar in everyday life – this is the simplest ‘buckled’ shape that an elastic ring can adopt, and has been understood for more than a century.
In recent years, buckling has begun to be recognized as a useful means of forming regular, periodic patterns in soft materials.
However, the wavelength of static buckle patterns, such as the figure-of-eight shape, is determined by material properties alone, which limits the versatility of buckling as a fabrication technique.
In a series of experiments, we show that dynamic buckling provides a way to override this intrinsic buckling wavelength so that different buckling patterns can emerge from the same system.
We floated an elastic band on a soap film, so that surface tension acts on the inside and outside of the band. Popping the outer soap film allowed the surface tension of the remaining, internal soap film to rapidly pull the band inwards. As the band collapsed in on itself, an intricate buckled shape formed.
Our results reveal that the number of buckles depends not only on material properties (i.e. stiffness and size of the elastic band) but also on the rate at which buckling happens, providing a method for controlling pattern formation.
This study of soapy, elastic bands could also be considered an elastic analogue of the Kibble-Zurek mechanism in cosmology, which describes how structure formation in the early universe depends on the rate of expansion following the Big Bang.
Read more about it here or on FYFD. Watch a video on the APS Gallery of Fluid Motion.
Dynamic buckling of an elastic ring in a soap film
F. Box, O. Kodio, D. O'Kiely, V. Cantelli, A. Goriely and D. Vella, Physical Review Letters 124, 198003 (2020) pdf
Cloaking by coating
From human tissue to fruits, many soft materials are coated by a thin layer of a stiffer material.
While the thin, stiff coating may be there to protect the softer material, it also affects the mechanical behaviour of the composite material.
So, what do we feel when we poke a cloaked substrate?
Well, that depends on the size of the poker: for indenters smaller than the lengthscale over which the composite deforms, the mechanical response is dominated by the bending of the thin coating; for indenters larger than this deformation lengthscale, we experience the stiffness (softness) of the underlying substrate.
It just so turns out that a finger is large enough to feel the flesh of most fruit via poking, rather than the skin, making it a suitable instrument for testing the ripeness of your 5-a-day!
Cloaking by coating: How effectively does a thin, stiff coating hide a soft substrate?
F. Box, C. Jacquemot, M. Adda-Bedia and D. Vella, Soft Matter 16, 4574-4583 (2020) pdf
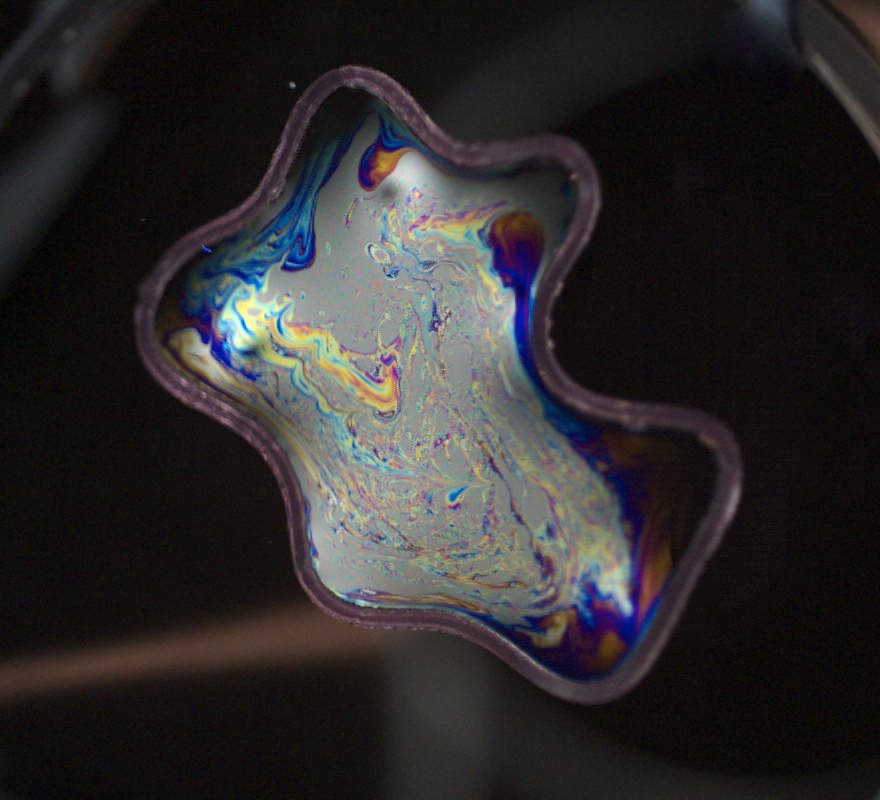
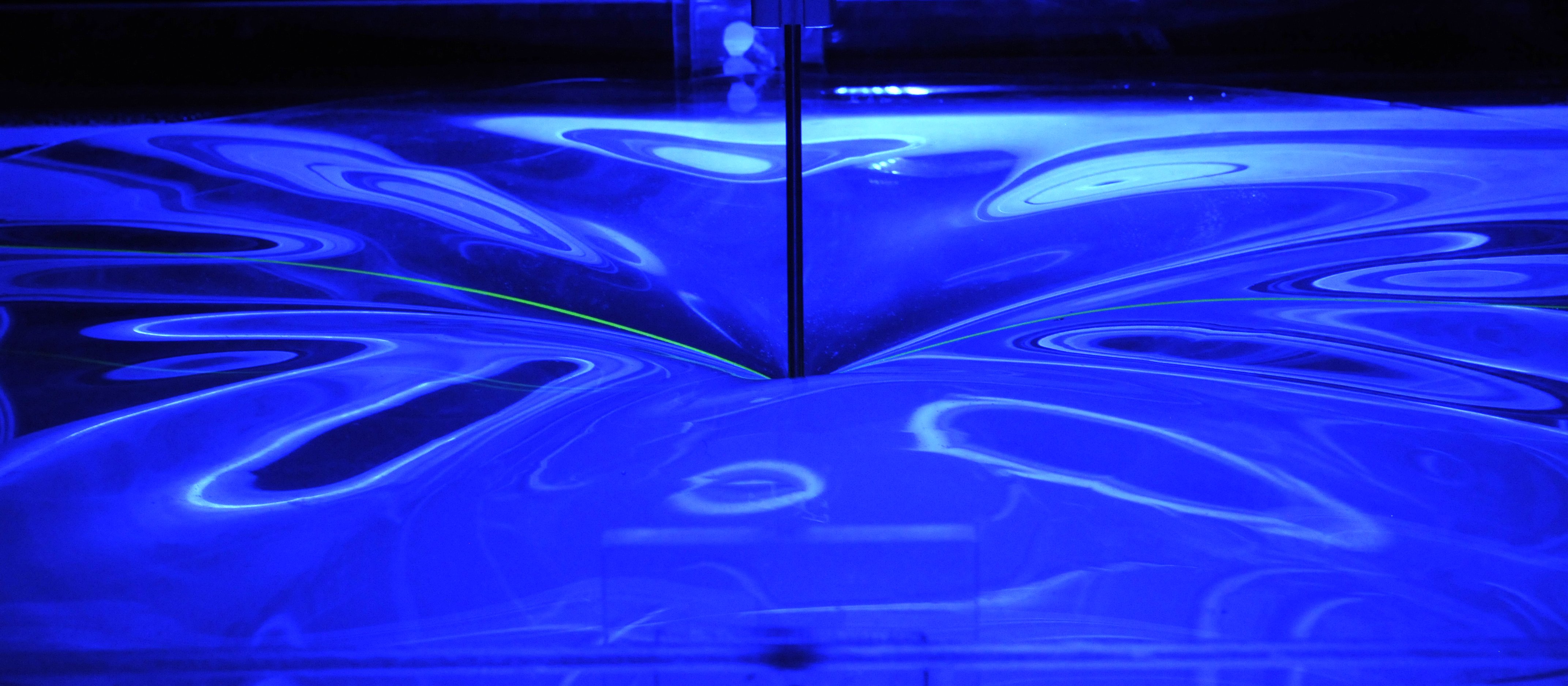
Dynamic wrinkling of ultrathin sheets
Elastic instabilities provide a means of generating regular topographies with a well-defined wavelength.
For example, a thin elastic film attached to a softer substrate buckles into an array of regular wrinkles under quasi-static compression.
The wrinkle wavelength is selected by the mechanical properties of the system, so that different wavelengths are typically attained through variation of the film thickness.
Dynamic wrinkling can generate evolving wrinkle wavelengths, however. For example, ballistic impact causes a floating sheet to retract inwards and displaces the fluid below.
The compression associated with this retraction results in a striking pattern of radial wrinkles, that evolves it time as fluid is moved.
This wrinkle coarsening is controlled by the inertia of the underlying fluid and raises the potential for dynamic substrate stiffness to be used as a means of breaking away from the single, static wavelength that is selected by material properties alone.
Read more about it here.
Dynamics of wrinkling in ultrathin elastic sheets
F. Box, D. O'Kiely, M. Inizan, O. Kodio, A. A. Castrejón-Pita, and D. Vella, Proceedings of the National Academy of Sciences 116(42), 201905755 (2019) pdf
Impact on floating thin elastic sheets: A mathematical model
D. O'Kiely, F. Box, O. Kodio, J. Whiteley and D. Vella, Physics of Fluids 5, 014003 (2020) pdf
Directed droplet transport on slippery surfaces
The Nepenthes pitcher plant employs a pitfall trapping mechanism to attract and digest animal prey; insects slide down the slippery, sloping peristome into a pool of digestive fluid from which they can't escape.
The peristome is slippery when wet, and has inspired new types of liquid-shedding surfaces, commonly know as Slippery Liquid-Infused Porous Surfaces (SLIPS).
Controlling the motion of droplets on SLIPS is inherently difficult because of a lack of drop-solid interaction.
Inspired by observations of ants and droplets sliding on the grooved peristome of a pitcher plant, we fabricated textured surfaces capable of trapping, retaining and directing the motion of liquid droplets;
the interfacial interaction between the droplet and lubricant layer acts to pin the droplet to a non-planar feature, or groove, favouring droplet motion along the feature to motion across it.
This discovery enables the design of textured, slippery surfaces that can
control the motion of sliding droplets, and provides insight into the functional evolution of the Nepenthes pitfall trap.
Read more about it here
or here.
Guided droplet transport on synthetic slippery surfaces inspired by a pitcher plant
F. Box, C. Thorogood and J. H. Guan , Journal of the Royal Society Interface 16(158), 20190323 (2019) pdf
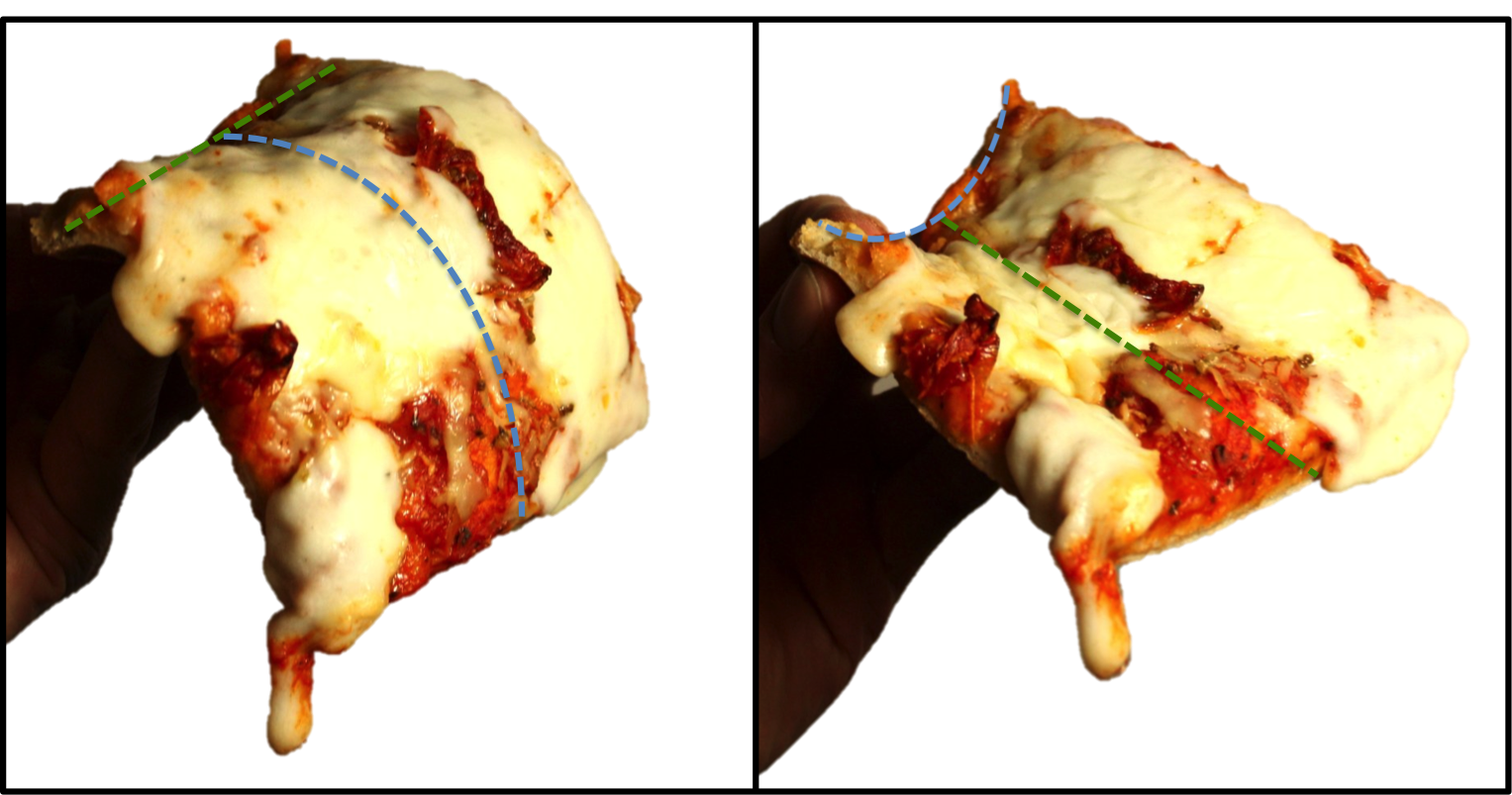
Gauss' pizzeria
The mechanics of pizza-eating are well known: curving the crust of a slice of pizza prevents it from drooping under its weight.
Insight into this intuitive solution to a meal-time conundrum comes from Gauss' Theorema Egregium, which states that changes in Gaussian curvature require an energetic cost associated with stretching.
Imposing curvature in the transverse direction induces a resistance to bending in the longitudinal direction since the sheet must then stretch in order to deform.
We explore the competition between curvature-induced rigidity and gravity-induced dropping, uncovering a catastrophic buckling phenomena with very messy consequences.
Limitations of curvature-induced rigidity: How a curved strip buckles under gravity
M Taffetani, F. Box, A Neveu, D Vella , Europhysics Letters 127(1), 14001 (2019) pdf
Deformation-enhanced capillary adhesion
Stick insects secrete fluid through the soles of their feet as they climb. Their adhesion to solid surfaces is therefore governed by capillary forces.
Does the fact their skin is soft and can deform make any differece to their sticking power?
Modelling the skin of a stick insect as a thin elastic sheet, we showed that deformation significantly enhances capillary adhesion, by orders of magnitude.
The surface tension of a drop pulls the 'skin' closer to the surface which, in turn, causes the drop to spread further, increasing the curvature of the drop meniscus and associated pinning force.
As this deformation-enhanced adhesion depends strongly on the tension in the sheet, we suggest that the stick insect could detach merely by pulling taut the skin of it's foot.
Beyond the sticky business of insect climbing, tension-controlled switchable adhesion could find application in soft robotics. Read more here
and here.
Elasto-capillary adhesion: The effect of deformability on adhesion strength and detachment
M. Butler, F. Box, T. Robert and D. Vella, Physical Review Letters 122, 07453 (2019) pdf
Bendotaxis - passive droplet transport
Fluid-structure interactions have typically been the reserve of engineers concerned by big buildings and fast flows.
Only in recent years have researchers began to investigate deformation induced by fluids at the small scale.
We demonstrated that by deforming thin, glass walls a droplet can self-propel along a flexible channel (click here to see a video).
The self-propulsion of the droplet is driven by a pressure gradient that results from the bending of the channel walls, induced by surface tension.
Perhaps surprisingly, the direction of droplet motion is independent wetting properties of the fluid - towards the free ends of the flexible channel.
This fluid-structure interaction could therefore be harnessed for functionality in the develoment of self-cleaning micro-mechanical devices.
Wettability-independent droplet transport by Bendotaxis
A. T. Bradley, F. Box, I. J. Hewitt, D. Vella, Physical Review Letters 122, 07453 (2019) pdf
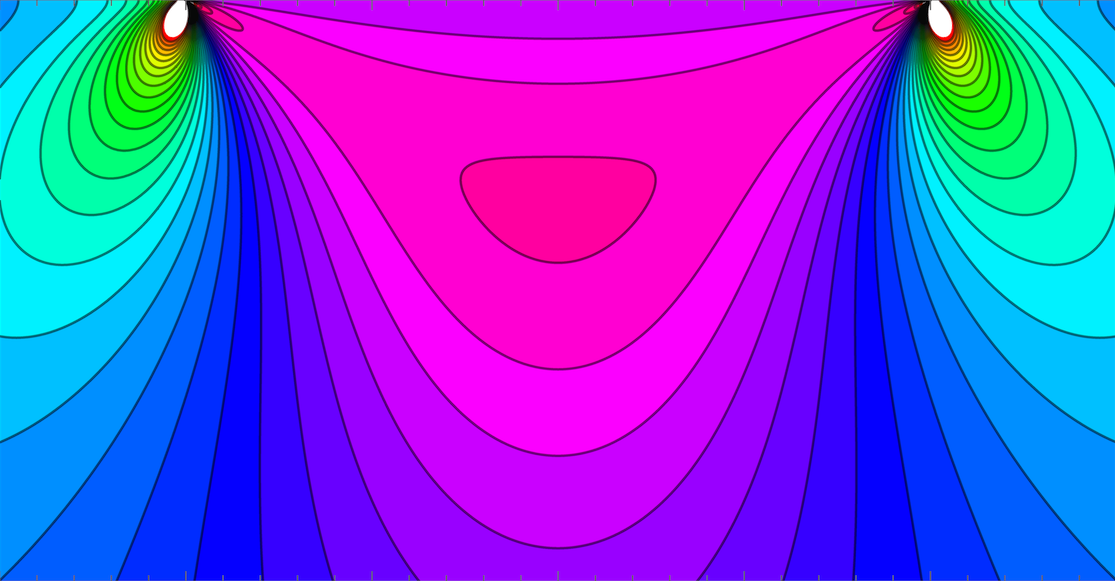
Bulging brains
Elastic solids bulge and swell in response to increases in internal pressure. A real-life example of this is the rise in intercranial pressure that occurs following traumatic brain injury. Decompressive craniectomy is a surgical procedure designed to relieve such pressure by removing part of the skull, so that the brain can bulge through the opening. We examined the influence that the boundary of the opening has on the stress and strain within a bulging elastic solid. The phsyical insight gained from our simplified model (of what is a highly complex physiological process) suggest that curving the edges of the opening can mitigate the build-up of dangerously large levels of stress.
Edge effects in elastic bulging
F. Benoist, F. Box and A. Goriely, International Journal of Non-linear Mechanics 106:227-237 (2018)
Poking floating sheets
Poking something is a natural way of testing its material properties. In everyday life, fruit is poked to check how ripe it is, and bicycles tyres to test how inflated they are.
Here, we poked a thin elastic sheet floating on a liquid bath and studied its resultant deformation. We mapped the transition from bending to stretching and investigated the influence that the surface tension of the liquid has on ultra-thin elastic membranes.
The findings are applicable across a broad spectrum of length scales, from AFM measurements of graphene membranes and biological membranes like skin, through frogs sitting on lily pads to mountain ranges that are supported by the Earth’s tectonic plates.
Indentation of a floating elastic sheet: geometry versus applied tension
F. Box, D. Vella, R. W. Style, and J. A. Neufeld, Proceedings of the Royal Society of London A 473:2206, (2017)
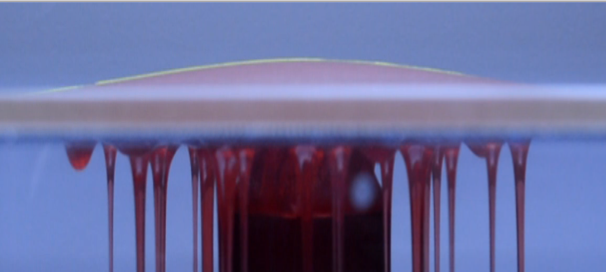
The leaky liquid blister
The spreading of a fluid in between the layers of a composite material is a fluid-structure interaction applicable to the formation of blisters under the skin and magmatic intrusions in between rock strata. Here, we investigated what happens when one of the layers is permeable so that the spreading fluid also drains away. We injected fluid in between an elastic sheet and a rigid, permeable substrate and measured the interaction between fluid flow and elastic deformation. We found that the draining of injection fluid through the permeable base arrests the propagation of the intruding fluid beneath the sheet, a finding which is applicable in hydraulic fracturing and CO2 sequestration.
Click here to see a video.
On the dynamics of a thin viscous film spreading in between an elastic sheet and a permeable horizontal substrate
F. Box, J. A. Neufeld and A. W. Woods, Journal of Fluid Mechanics 841, 989-1011 (2018)
Manufacturing micro-robots
Counter-intuitive to our experience of propulsion in water, swimming in a very viscous fluid is a difficult feat as viscosity dominates over inertia, rendering motion reversible in time; the forward-backward motion of a paddle won't get you anywhere when your a small creature in a sticky biofluid.
Evolution has engineered ingenious solutions to the problem of time-reversibility enabling micro-organisms to push and pull themselves along - an pertinent example is the helical motion of the spermatoza tail.
Humankind has been slower to engineer solutions to this sticky problem, however.
During my PhD I developed a magnetically-actuated device capable of swimming in biofluids.
The swimming device comprises an array of spheres attached by elastic tethers; the motion of the spheres is remotely controlled by an applied magnetic field and a geometrical asymmetry built into the design generates a non-reciprocal motion that overcomes time-reversibility, resulting in self-propulsion.
As the device is driven by an oscillatory field, it can transport cargo over much larger distances than is currently achieved by biomedical magnetic-guidance systems, which instead use field gradients to transport magnetic particles.
Using nanorobots for targeted drug delivery may remain a futuristic possibility, but it is a futuristic possibility that is one sticky step closer than before.
On the motion of linked spheres in a Stokes flow
F. Box, E. Han, C. Tipton and T. Mullin, Experiments in Fluids 58:29 (2017) pdf
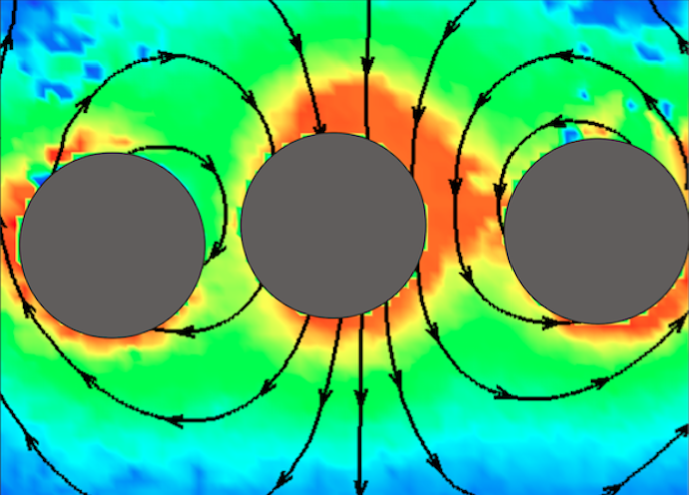
Dancing balls in viscous fluids
The motion of particles in a fluid is an ubiquitous process found throughout nature and industry.
Many applications exist in which particles are dispersed in a fluid, from paints and polymer suspensions to turbidity currents which distribute and deposit sediment in the deep ocean.
Investigating the motion of particle in a fluid without affecting the local environment is a challenge.
In this research, we developed a novel technique to control the oscillatory motion of a sphere immersed in a fluid that is simple and cheap to implement experimentally.
We then used this non-contact method to investigate the influence that nearby solid boundaries have on an oscillating particle in a very viscous fluid and observed how a driven sphere moves a second sphere in its vicinity.
The interaction between oscillating spheres and solid boundaries at low Reynolds number
F. Box, K. Singh and T. Mullin, Journal of Fluid Mechanics 849:834-859 (2018) pdf
Torsional oscillations of a sphere in a Stokes flow
F. Box, A. B. Thompson and T. Mullin, Experiments in Fluids 56:209 (2015) pdf
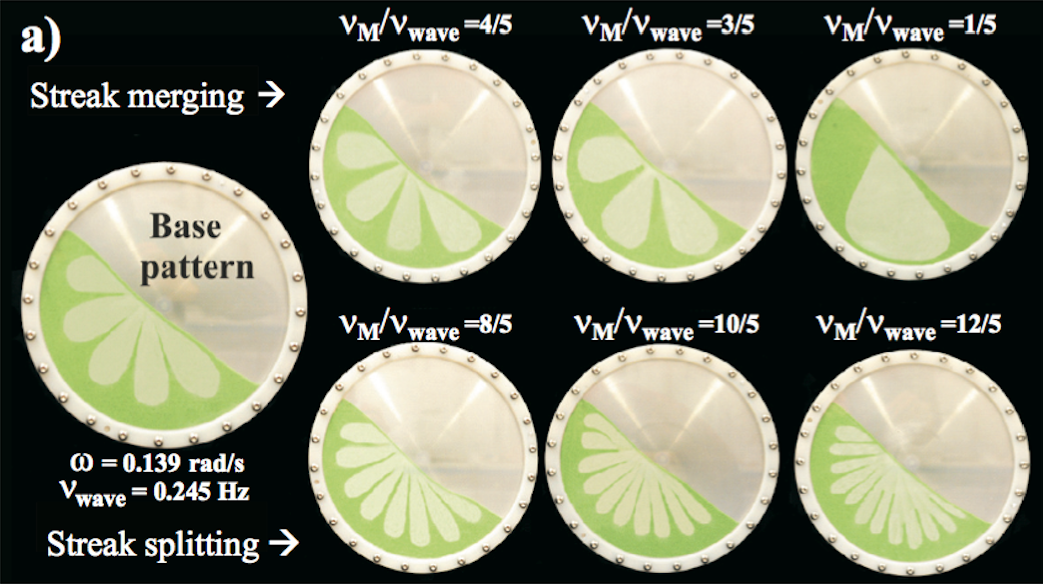
Granular flowers
Segregation of granular materials occurs in industrial silos and in everyday life when smaller pieces fall to the bottom of the cereal packet - a process commonly known as the ‘Brazil nut effect’. We explored the segregation of a binary mixture of particles in a thin rotating drum. Slow rotation of the drum caused the particles to segregate in a radial pattern that resembles petals. The number of petals which form depends on the rate at which the drum is rotated. By modulating the rotation rate we could induce a change in the number of granular petals compared to the number which form for steady rotation. Increases in the number of petals occurred through petal splitting, while decreases occurred through petal merging.
Granular segregation in a thin drum rotating with periodic modulation
R. D. P. East, P. McGuiness, F. Box, T. Mullin and I. Zuriguel, Physical Review E 90, 052205 (2014)
Bubble propagation
The formation of bubbles sneaking in tubes can be an inconvenience in flow delivery systems, yet understanding the propagation of bubbles in tubes is of serious biomedical importance. When a newborn baby draws its first breath, air is sucked into its lungs through fluid-filled tubes. The shape the bubble takes as it travels into the lungs is important in determining how effectively the collapsed airways reopen. In this research we showed that the geometry of the tube alone can influence the shape of the propagating bubble. Partial occlusion of a rectangular channel can cause a switch in shape, from symmetric bubbles to asymmetric bubbles that propagate in the least-constricted regions of the tube. The switchlike transition in bubble type occurs abruptly at a critical flow rate and provides a powerful means of controlling bubble traffic in microfluidic systems.
Tube geometry can force switchlike transitions in the behavior of propagating bubbles
Physics of Fluids 21, 101702 (2009)
A. de Lózar, A. Heap, F. Box, A. L. Hazel, and A. Juel