Team
Sergei Fedotov
Professor of Applied Mathematics
Nickolai Korabel
Post-doctoral researcher, supported by EPSRC grants EP/008641/1
Anomalous transport and fractional PDEs in physics & biology
We are a research group based in the School of Mathematics in The University of Manchester, UK.
Our research is supported by
FAPESP-UoM grant: Random walks with strongly correlated memory and applications to biology 2018-2021. Value: £40,000
EPSRC grant EP/v008641/1: Modelling anomalous transport of nanoparticles and DNA repair to improve radiotherapy 2021-2024. Value: £702,576
EPSRC grant EP/S024344/1: BioProton: Biologically relevant dose for Proton Therapy Planning, 2019-2024. Value: £1,394,633
EPSRC grant EP/N018060/1: Non-Markovian models of intracellular transport in a heterogeneous environment, 2016-2019. Value: £371,157
EPSRC grant EP/J019526/1: Anomalous reaction-transport equations, 2012-2016. Value: £291,257
What is anomalous transport?
This is a new, exciting area of research because it is a widespread natural phenomenon. Examples include flight of albatross, human migration, movement of proteins on cell membranes and signalling molecules in the brain, transport on social networks and fractal geometries, bacterial motility. Anomalous transport cannot be described by standard tools like diffusion equation. Instead it requires the use of fractional partial differential equations involving fractional derivatives of non-integer order. Diffusion processes in complex systems may fail to obey linear law for unforced diffusion \( \langle x^2(t) \rangle \sim t \). They may instead obey a power law pattern of growth of the mean squared displacement \( \langle x^2(t) \rangle \sim t^\gamma \).
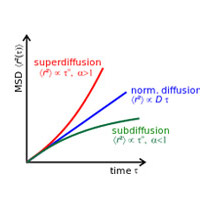
Anomalous subdiffusive systems observe ultra-slow transport with an evolution of mean squared displacement as \( \langle x^2(t) \rangle \sim t^\gamma \) for \( 0 < \gamma < 1 \). The relaxation is governed by the Mittag-Leffler function, which interpolates between an initial stretched exponential and asymptotic power law. The Mittag-Leffler function is an exact solution of the fractional relaxation equation \( \frac{d \Phi(t)}{dt} = - D_t^{1-\gamma} \Phi(t) \), where \( D_t^{1-\gamma} \) is the Riemann-Liouville fractional derivative operator.
Anomalous diffusion is an observed natural phenomenon, found in many varied areas of science including: transport of lipids on cell membranes, bacterial motion, transport on fractal geometries, NMR diffusometry in porous systems, tracer diffusion in subdiffusive hydrology, signalling molecules in spiny dendrites, RNA molecules in cells, flight of albatross, financial futures prices, quantum optics, dispersive transport in amorphous semiconductors, and others.
Subdiffusion is classically described in terms of the continuous time random walk (CTRW) with an asymptotic power law waiting time of the form \( \psi(t) \sim \tau_0 / t^{1 + \gamma} \) for \( 0 < \gamma < 1 \) and \( t \gg \tau \). This is characterised by a diverging first moment, ie. an infinite mean waiting time for walkers. Such waiting times introduce deeply non-Markovian behaviour into the CTRW, where the current state of the system now depends on the entire history from preparation.
From the CTRW model with anomalously long waiting times one can recover, in the diffusion limit, the fractional Fokker-Planck equation for position, time pdf \( p(x,t) \) $$ \frac{\partial p}{\partial t} =\mathcal{D}_t^{1-\gamma}K_\gamma\left[ \frac{\partial^2}{\partial x^2}-\frac{\partial }{\partial x}\frac{F(x)}{k_BT}\right]p(x,t)$$
What do we do?
.
We are interested in
.
.
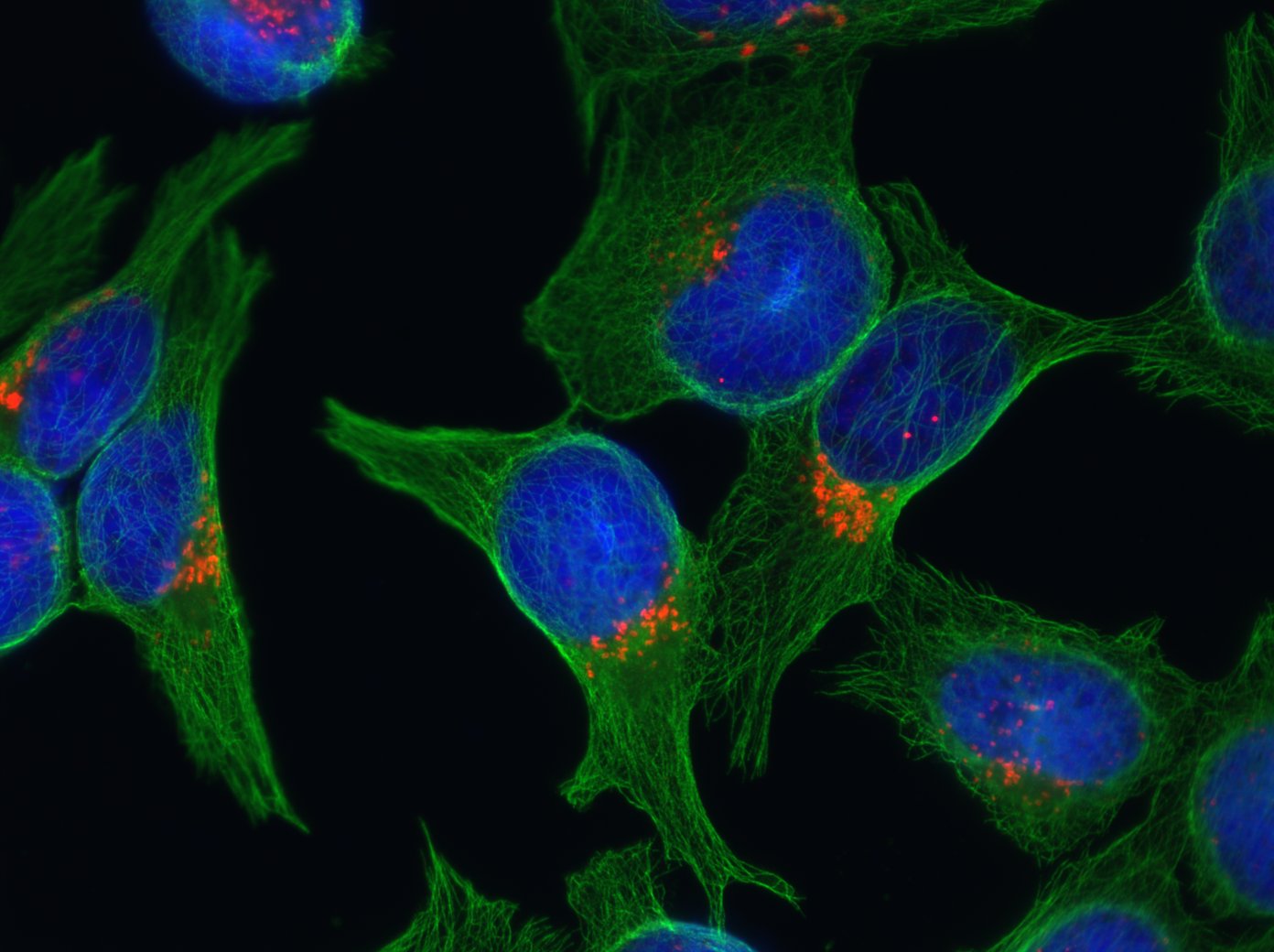
We are studying the transport of all kinds of components within the cell - from vesicles along the cytoskeleton through to transcription factors along DNA. Despite experimental facts that intracellular transport is heterogeneous and non-Markovian with subdiffusive and superdiffusive regimes most mathematical models for vesicles trafficking are Markovian and homogeneous. The main challenge for our Manchester interdisciplinary team is to obtain new non-Markovian models of heterogeneous intracellular transport supported by experiments. These models will provide a tool set for analysing transport processes in a much more realistic way, opening the way for greatly improved analysis and ultimately understanding of these highly complex cellular behaviours. This will allow other researchers to formulate and test new hypotheses. In the long term, therefore, non-Markovian models have the potential to lead to insight into neurological diseases, ageing and other processes that involve intracellular transport such as bacterial and viral infection. Such knowledge will be important for developing new treatments. Our project combines three different approaches: mathematical modelling, numerical modelling and experimental validation, which complement each other. This strategy will provide multidisciplinary study of the intracellular transport problem and ensure maximum impact across and within several disciplines.
We are also interested in the study of chemotaxis, anomalous aggregation within a subdiffusive environment, where the diffusion is influenced by a gradient of concentration of an external chemical signal. In inhomogeneous media, under the CTRW framework, the escape rate of a random walker from a point is not constant throughout the domain. This introduces a dependence of the anomalous exponent on the spatial co-ordinate. Any spatial fluctuation in the anomalous exponent can lead to the phenomenon of anomalous aggregation. Anomalous aggregation can be negated by a tempering of the anomalous waiting time. We are interested in studying the interaction between attractive forces such as anomalous aggregation, and possible repulsive forces such as nonlinear reactions and chemotactic forces.
