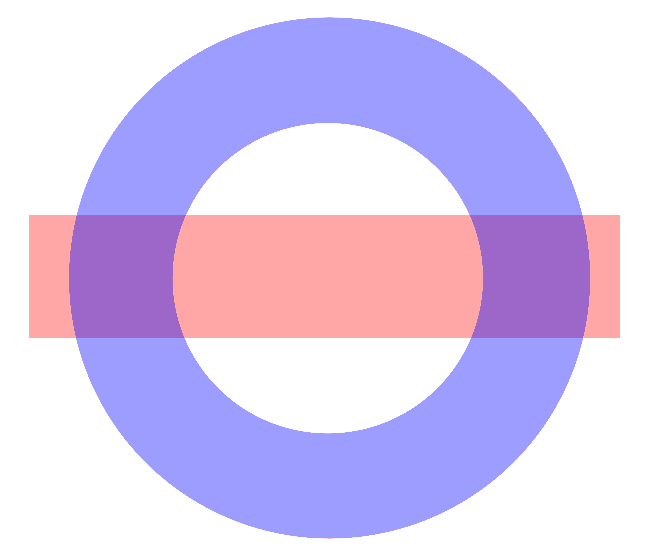
The red and blue sets above are both domains. However, their intersection is not path connected. The intersection of two domains need not be a domain.
Home | Assessment | Notes | Index | Worksheets | Blackboard
Fix $1 \le c \le 2$. We calculate \begin{align*} \lim_{t \to c} \gamma(t) & = \lim_{t \to c} 4t^2 + 2it \\ & = 4 \lim_{t \to c} t^2 + 2i \lim_{t \to c} t \\ & = 4c^2 + 2ic = \gamma(c) \end{align*} using the limit laws, which proves $\gamma$ is continuous at $c$. Since $1 \le c \le 2$ was arbitrary $\gamma$ is continuous on $[1,2]$.
Take \[ \gamma(t) = (1-t)2 + t(i-1) = 2 + t(i-3) \] which is continuous and satisfies has $\gamma(0) = 2$ and $\gamma(1) = i-1$.

The red and blue sets above are both domains. However, their intersection is not path connected. The intersection of two domains need not be a domain.
Write $z = x+iy$. For $f(z) = \Re(z)$ we have $u(x,y) = x$ and $v(x,y) = 0$. For \[ f(z) = |z| = \sqrt{x^2 + y^2} \] we have $u(x,y) = \sqrt{x^2 + y^2}$ and $v(x,y) = 0$.
Calculate \[ \begin{align*} & (\nabla u)(x,y) \bullet (\nabla v)(x,y) \\ ={} & \langle 2x, -2y \rangle \bullet \langle 2y, 2x \rangle \\ ={} & (2x)(2y) + (-2y)(2x) \\ ={} & 0 \end{align*} \]
With $z = t$ we have \[ \lim\limits_{t \to 0} \dfrac{|t|}{t} = 1 \] and with $z = it$ we have \[ \lim\limits_{t \to 0} \dfrac{|it|}{it} = -i \] so the complex limit does not exist.
Here $\left| \dfrac{|z|^2}{z} \right| = |z|$ which does go to zero as $z \to 0$.
With $z = t > 0$ we have \[ \lim\limits_{t \to 0} \Arg(t) = 0 \] while with $z = it$ we have \[ \lim\limits_{t \to 0} \Arg(t) = \dfrac{\pi}{2} \] so the complex limit does not exist.
One can use the fact that $|\Re(z) - \Re(a)| \le |z-a|$ to prove \[ \lim\limits_{z \to a} \Re(z) = \Re(a) \] for all $a \in \C$. Therefore $f$ is continuous on $\C$.
Use the reverse triangle inequality $||z| - |a|| \le |z-a|$ to prove that \[ \lim\limits_{z \to a} |z| = |a| \] holds.
Fix $a \in \C \setminus (-\infty,0]$ and put $\theta = \Arg(a)$.