Week 1 Worksheet - Solutions
Home | Assessment | Notes | Index | Worksheets | Blackboard
Complex Numbers
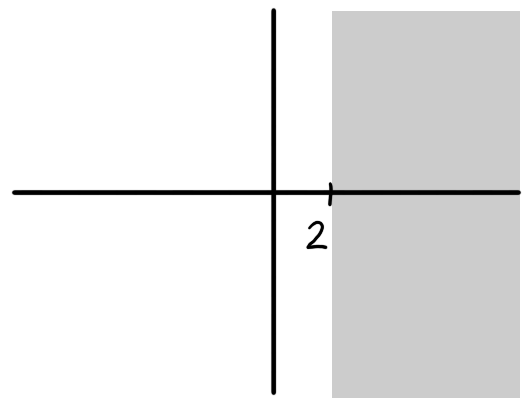
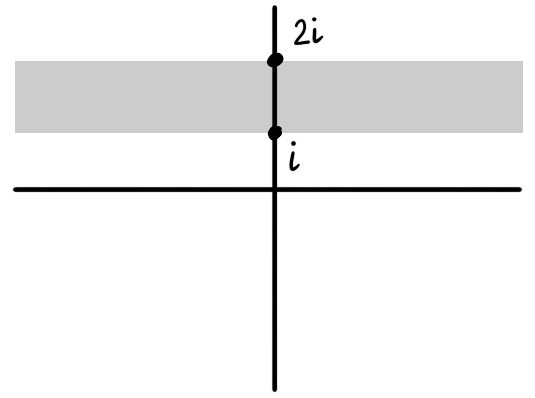
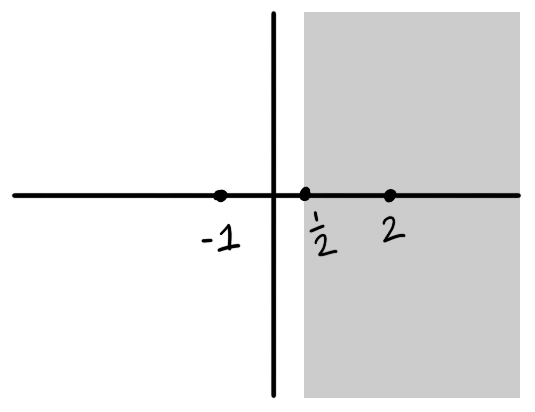
- . The arguments are for any . .
- . The arguments are for any . The principal argument is .
- . The arguments are for any . .

Arithmetic
-
Calculate using the rules of arithmetic.
- (becuase )
Write so that the equation becomes . Real and imaginary parts must be equal, so and . By inspection we can take or . The solutions are and .
Completing the square gives so . Writing gives the equations and . By inspection, we can take or . The solutions are therefore and .
-
Calclate
-
Calculate
Take . Then
but . Also but .
-
Calcuate
-
Calcuate
-
Calcuate
The case is immediate. We assume
and calculate
because when we multiply complex numbers we add arguments and multiply moduli.
We have
so equating real and imaginary parts tell us
and
hold.
From
we get also that
and
hold.
Write . If then and is an argument of . Thus for some . This means
for some . Taking gives all possible distinct as after that we repeat.
Take . We have . But
has principal argument .
Sequences
- Fix . There is such that guarantees . If then by the reverse triangle inequality.
- does not converge, so does not converge.
Calculate
and note that if is large enough then so which does not converge, so does not converge.
- converges to 0 so converges to 0.
- There is such that for all . Now for we have
which converges to zero as .
Series
- Let's look at the partial sums. Write where . Then
is either , , or . That is
and the partial sums do not converge.
- From we can apply the geometric series formula to get
as the limit. (We subtract 1 because the series began at .)
- Yes, but this is not easy to prove from the tools we have, because we cannot compare this series with the geometric series. (The ratio and root tests are not conclusive.) We can write
so that
is increasing and bounded. Therefore the partial sums converge.
- For every we have
so the statement is a consequence of our theorem about convergence of sequences in terms of their real and imaginary parts.